Royal-flush twice in a row. What is the probability?

[BelFish]

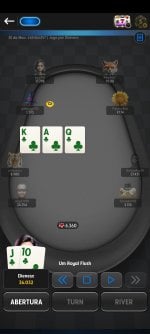
The probability of getting a royal flush in Texas Hold'em if people didn't fold preflop and postflop some hands would be:

P = 4*C(5 of 5)*C(2 of 47)/C(7 of 52)=4324/133784560=0.0000323 or 0.00323%

Taking into account folds, we will roughly estimate this probability as a number of 0.00001, or 1 time for every 100,000 hands.

Then the probability of getting a 2 royal flush in the next 2 hands will be equal to 1 in 10 billion hands.

And here is that complex formula for calculating at a distance of N hands (for calculation in excel):

A1 is P
A2 is K
A3 is N
A6 is (p), or the probability of the event for the repeat series we are looking for.

A4=1+(1-A1)*A1^A2+(A2+1)*(1-A1)^2*A1^(2*A2)

A5=((1-A1*A4)/((A2+1-A2*A4)*(1-A1)))/A5^(A3+1)

A6=1-A5

Here is an example of calculating this formula for playing roulette: the probability that 11 or more times in a row will be one color, for example, red at a distance of 2000 games, is 47%, and for a distance of 5000 games this probability will be equal to 80%

Here we substitute K=11, P=18/37 into the formula and calculate for cases N=2000 and N=5000

K is the number of repetitions of the event in a row in a series of N games, and P is the probability of this event.

Similarly, using the same formula, you can calculate the probability of getting a royal flush 2 times in a row at any distance. To do this, you need to substitute K=2, P=0.00001 into the formula and see what happens for different values of N (1000, 10000, 100000, 10000000)

 

[0546474]

I have been playing poker for money since 2015 and during this time I have collected a Royal flash combination many times, but I have never seen this combination collected twice in a row !!! This is indeed a very rare case!

 

[azteca6]

I never believe it once a month and if you're lucky: D

 

[Recreationalplayer]

Its a good fantasy to be in awe when you make such hands.

But most of the winnings come from Top pair Top Kicker and set mining.

In the long run that's what matters.

 

[IronMike9000]

When i shuffle the cards i get dealt the royal flush every time, however from my experience other players start to get suspicious around the 6th royal flush in a row

 

[Djon25]

Poker rooms are greedy, they could give some prizes for the collected royal flush.

 

[SL-247]

Life is so short that the chance like this will never happen.

 

[luckyfish98]

Royal-flush twice in a row. What is the probability? but losing 10 years from a place is also not possible, that is the positive, but the negative happens much more in poker games

 

[aissam18]

To be honest you can get hit by a meteor than to have royal flush 2 times in a row

 

[nakiesQUensyyy]

I think about zero if 2 in a row, I won’t believe if a person catches him, I never saw how much I play and never caught it myself

 

[iceheart888]

In the early years of online poker, it was difficult to see it twice in one year, now there is nothing surprising

 

[NightStalker]

I prefer to win the lottery.

 

[andron205]

very very small